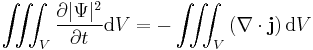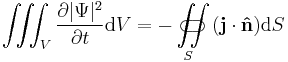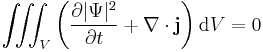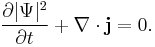Continuity equation
A continuity equation in physics is an equation that describes the transport of a conserved quantity. Since mass, energy, momentum, electric charge and other natural quantities are conserved under their respective appropriate conditions, a variety of physical phenomena may be described using continuity equations.
Continuity equations are the (stronger) local form of conservation laws. All the examples of continuity equations below express the same idea, which is roughly that: the total amount (of the conserved quantity) inside any region can only change by the amount that passes in or out of the region through the boundary. A conserved quantity cannot increase or decrease, it can only move from place to place.
Any continuity equation can be expressed in an "integral form" (in terms of a flux integral), which applies to any finite region, or in a "differential form" (in terms of the divergence operator) which applies at a point.
Contents |
General equation
Preliminary description
As stated above, the idea behind the continuity equation is the flow of some property, such as mass, energy, charge, momentum, and even probability, through surfaces from one region of space to another. The surfaces, in general, may either be open or closed, real or imaginary, and have an arbitrary shape, but are fixed for the calculation (i.e. not time-varying, which is appropriate since this complicates the maths for no advantage). Let this property be represented by just one scalar variable, q, and let the volume density of this property (the amount of q per unit volume V) be φ, and the all surfaces be denoted by S. Mathematically, φ is a ratio of two infinitesimal quantities:
which has the dimension [quantity][L]-3.
There are different ways to conceive the continuity equation:
- either the flow of particles carrying the quantity q, described by a velocity field v, which is also equivalent to a flux f of q (a vector function describing the flow per unit area per unit time of q), or
- in the cases a velocity field is not useful or applicable, the flux f of the quantity q only (no association with velocity).
In each of these cases, the transfer of q occurs as it passes through two surfaces, the first S1 and the second S2.
The flux f should represent some flow or transport, which has dimensions [quantity][T]−1[L]−2. In cases where particles/carriers of quantity q are moving with velocity v, such as particles of mass in a fluid or charge carriers in a conductor, f can be related to v by:
This relation is only true in situations where there are particles moving and carrying q - it can't always be applied. To illustrate this: if f is electric current density (electric current per unit area) and φ is the charge density (charge per unit volume), then the velocity of the charge carriers is v. However - if f is heat flux density (heat energy per unit time per unit area), then even if we let φ be the heat energy density (heat energy per unit volume) it does not imply the "velocity of heat" is v (this makes no sense, and is not practically applicable). In the latter case only f (with φ) may be used in the continuity equation.
Elementary vector form
Consider the case when the surfaces are flat and planar cross-sections. For the case a velocity field can be applied, dimensional analysis leads to this form of the continuity equation:
where
- the left hand side is the initial amount of q flowing per unit time through surface S1, the right hand side is the final amount through surface S2,
- S1 and S2 are the vector areas for the surfaces S1 and S2 respectively.
Notice the dot products 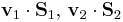 are volumetric flow rates of q. The dimension of each side of the equation is [quantity][L]-3·[L][T]-1·[L]2 = [quantity][T]-1. For the more general cases, independent of whether a velocity field can be used or not, the continuity equation becomes:
are volumetric flow rates of q. The dimension of each side of the equation is [quantity][L]-3·[L][T]-1·[L]2 = [quantity][T]-1. For the more general cases, independent of whether a velocity field can be used or not, the continuity equation becomes:
This has exactly the same dimensions as the previous version. The relation between f and v allows us to pass back to the velocity version from this flux equation, but not always the other way round (as explained above - velocity fields are not always applicable). These results can be generalized further to curved surfaces by reducing the vector surfaces into infinitley many differential surface elements (that is S → dS), then integrating over the surface:
more generally still:
in which
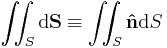 denotes a surface integral over the surface S,
denotes a surface integral over the surface S,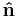 is the outward-pointing unit normal to the surface S
is the outward-pointing unit normal to the surface S
N.B: the scalar area S and vector area S are related by 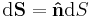 . Either notations may be used interchangeably.
. Either notations may be used interchangeably.
Differential form
The differential form for a general continuity equation is (using the same q, φ and f as above):
where
- ∇∙ is divergence,
- t is time,
- σ is the generation of q per unit volume per unit time. Terms that generate (σ > 0) or remove (σ < 0) q are referred to as a "sources" and "sinks" respectively.
This general equation may be used to derive any continuity equation, ranging from as simple as the volume continuity equation to as complicated as the Navier–Stokes equations. This equation also generalizes the advection equation. Other equations in physics, such as Gauss's law of the electric field and Gauss's law for gravity, have a similar mathematical form to the continuity equation, but are not usually called by the term "continuity equation", because f in those cases does not represent the flow of a real physical quantity.
In the case that q is a conserved quantity that cannot be created or destroyed (such as energy), this translates to σ = 0, and the continuity equation is:
Integral form
By the divergence theorem (see below), the continuity equation can be rewritten in an equivalent way, called the "integral form":
where
- S is a surface as described above - except this time it has to be a closed surface that encloses a volume V,
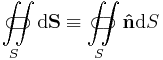 denotes a surface integral over a closed surface,
denotes a surface integral over a closed surface, denotes a volume integral over V.
denotes a volume integral over V.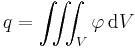 is the total amount of φ in the volume V;
is the total amount of φ in the volume V;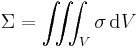 is the total generation (negative in the case of removal) per unit time by the sources and sinks in the volume V,
is the total generation (negative in the case of removal) per unit time by the sources and sinks in the volume V,
In a simple example, V could be a building, and q could be the number of people in the building. The surface S would consist of the walls, doors, roof, and foundation of the building. Then the continuity equation states that the number of people in the building increases when people enter the building (an inward flux through the surface), decreases when people exit the building (an outward flux through the surface), increases when someone in the building gives birth (a "source" where σ > 0), and decreases when someone in the building dies (a "sink" where σ < 0).
Derivation and equivalence
The differential form can be derived from first principles as follows.
Derivation of the differential form
Suppose first an amount of quantity q is contained in a region of volume V, bounded by a closed surface S, as described above. This is equal to the amount in already in V, plus the generated amount s (total - not per unit time or volume):
where s is such that:
The rate of change of q leaving the region is simply the time derivative:
where the minus sign has been inserted since the amount of q is decreasing in the region. (Partial derivatives are used since they enter the integrand, which is not only a function of time, but also space due to the density nature of φ - differentiation needs only to be with respect to t). The rate of change of q crossing the boundary and leaving the region is:
so equating each expression for 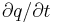 :
:
Using the divergence theorem on the left-hand side:
This is only true if the integrands are equal, which directly leads to the differential continuity equation:
Either form may be useful and quoted, both can appear in hydrodynamics and electromagnetism, but for quantum mechanics and energy conservation, only the first may be used. Therefore the first is more general.
Equivalence between differential and integral form
Starting from the differential form which is for unit volume, multiplying throughout by the infinitesimal volume element dV and integrating over the region gives the total amounts quantities in the volume of the region (per unit time):
again using the fact that V is constant in shape for the calculation, so it is independent of time and the time derivatives can be freely moved out of that integral, ordinary derivatives replace partial derivatives since the integral becomes a function of time only (the integral is evaluated over the region - so the spatial variables become removed from the final expression and t remains the only variable).
Using the divergence theorem on the left side obtains the integral form:
Equivalence between elementary and integral form
Starting from
the surfaces are equal (since there is only one closed surface), so S1 = S2 = S and we can write:
The left hand side is the flow rate of quantity q occurring inside the closed surface S. This must be equal to
since some is produced by sources, hence the positive term Σ, but some is also leaking out by passing through the surface, implied by the negative term -dq/dt. Similarly the right hand side is the amount of flux passing through the surface and out of it, so
Equating these obtains the integral form:
Electromagnetism
3-currents
In electromagnetic theory, the continuity equation can either be regarded as an empirical law expressing (local) charge conservation, or can be derived as a consequence of two of Maxwell's equations. It states that the divergence of the current density J (in amperes per square meter) is equal to the negative rate of change of the charge density ρ (in coulombs per cubic metre),
Maxwell's equations are a quick way to derive the continuity of charge.
One of Maxwell's equations, Ampère's law (with Maxwell's correction), states that
Taking the divergence of both sides results in
but the divergence of a curl is zero, so that
Another one of Maxwell's equations, Gauss's law, states that
substitution into the previous equation yields the continuity equation
4-currents
Conservation of a current (not necessarily an electromagnetic current) is expressed compactly as the Lorentz invariant divergence of a four-current:
where
- c is the speed of light
- ρ; the charge density
- j the conventional 3-current density.
- μ; labels the space-time dimension
since
then
which implies that the current is conserved:
Interpretation
Current is the movement of charge. The continuity equation says that if charge is moving out of a differential volume (i.e. divergence of current density is positive) then the amount of charge within that volume is going to decrease, so the rate of change of charge density is negative. Therefore the continuity equation amounts to a conservation of charge.
Fluid dynamics
In fluid dynamics, the continuity equation states that, in any steady state process, the rate at which mass enters a system is equal to the rate at which mass leaves the system.[1][2] In fluid dynamics, the continuity equation is analogous to Kirchhoff's current law in electric circuits.
The differential form of the continuity equation is:[1]
where
- ρ is fluid density,
- t is time,
- u is the flow velocity vector field.
If ρ is a constant, as in the case of incompressible flow, the mass continuity equation simplifies to a volume continuity equation:[1]
which means that the divergence of velocity field is zero everywhere. Physically, this is equivalent to saying that the local volume dilation rate is zero.
Further, the Navier-Stokes equations form a vector continuity equation describing the conservation of linear momentum.
Energy
By conservation of energy, which can only be transferred and not created or destroyed leads to a continuity equation, an alternative mathematical statement of energy conservation to the thermodynamic laws.
Letting
- u = local energy density (energy per unit volume),
- q = energy flux (transfer of energy per unit cross-sectional area per unit time) as a vector,
the continuity equation is:
Quantum mechanics
In quantum mechanics, the conservation of probability also yields a continuity equation. The terms in the equation require these definitions, and are slightly less obvious than the other forms of volume densities, currents, current densities etc., so they are outlined here:
- The wavefunction Ψ for a single particle in the position-time space (rather than momentum space) - i.e. functions of position r and time t, Ψ = Ψ(r, t) = (x, y, z, t).
- The probability density function ρ = ρ(r, t) is:
- The probability that a measurement of the particle's position will yield a value within V at t, denoted by P = Pr ∈ V(t), is:
- The probability current (aka probability flux) j:
With these definitions the continuity equation reads:
Either form is usually quoted. Intuitively; the above quantities indicate this represents the flow of probability. The chance of finding the particle at some r t flows like a fluid, the particle itself does not flow deterministically in the same vector field.
For this derivation see for example.[3] The 3-d time dependent Schrödinger equation and its complex conjugate (i → –i) throughout are respectively:
where U is the potential function. The partial derivative of ρ with respect to t is:
Multiplying the Schrödinger equation by Ψ* then solving for 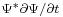 , and similarly multiplying the complex conjugated Schrödinger equation by Ψ then solving for
, and similarly multiplying the complex conjugated Schrödinger equation by Ψ then solving for 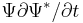 ;
;
substituting into the time derivative of ρ:
The Laplacian operators (∇2) in the above result suggest that the right hand side is the divergence of j, and the reversed order of terms imply this is the negative of j, altogether:
so the continuity equation is:
The integral form follows as for the general equation.
The time derivative of P is
where the last equality follows from the product rule and the fact that the shape of V is fixed for the calculation and therefore independent of time - i.e. the time derivative can be moved through the integral. To simplify this further consider again the time dependent Schrödinger equation and its complex conjugate, in terms of the time derivatives of Ψ and Ψ* respectively:
Substituting into the preceding equation:
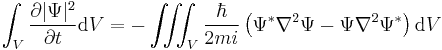 .
.
From the product rule for the divergence operator
substituting:
On the right side, the argument of the divergence operator is j,
using the divergence theorem again gives the integral form:
To obtain the differential form:
The differential form follows from the fact that the preceding equation holds for all V, and as the integrand is a continuous function of space, it must vanish everywhere:
See also
- Conservation law
- Euler equations
- Groundwater energy balance
- Incompressible fluid
- Mass flow rate
- Noether's Theorem
- Probability density function
- Schrödinger equation
References
Further reading
- Hydrodynamics, H. Lamb, Cambridge Univresity Press, (2006 digitalization of 1932 6th edition) ISBN 13-978-0-521-45868-9
- Introduction to Electrodynamics (3rd Edition), D.J. Griffiths, Pearson Education Inc, 1999, ISBN 81-7758-293-3
- Electromagnetism (2nd edition), I.S. Grant, W.R. Phillips, Manchester Physics Series, 2008 ISBN 0 471 92712 0
- Gravitation, J.A. Wheeler, C. Misner, K.S. Thorne, W.H. Freeman & Co, 1973, ISBN 0-7167-0344-0
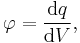
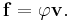


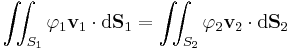


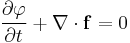

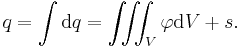
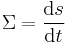
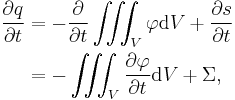
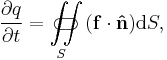

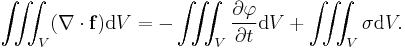
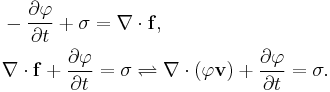
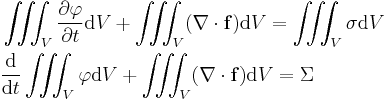



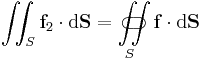
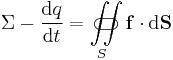
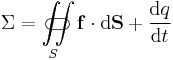
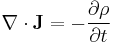
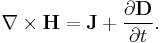
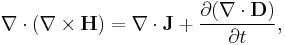
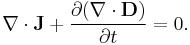
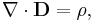
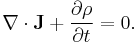
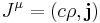
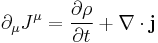
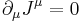
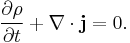
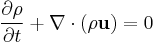
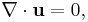
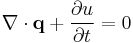
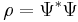
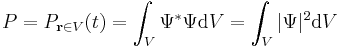
![\mathbf{j} = \frac{\hbar}{2mi} \left [ \Psi^{*} \left ( \nabla \Psi \right ) - \Psi \left ( \nabla \Psi^{*} \right ) \right ].](/2012-wikipedia_en_all_nopic_01_2012/I/bde9dc92855b78f5ee62f50445a5bd6e.png)
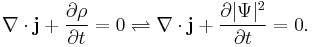
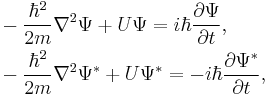
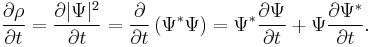
![\begin{align} & \Psi^*\frac{\partial \Psi}{\partial t} = \frac{1}{i\hbar } \left [ -\frac{\hbar^2\Psi^*}{2m}\nabla^2 \Psi %2B U\Psi^*\Psi \right ], \\
& \Psi \frac{\partial \Psi^*}{\partial t} = - \frac{1}{i\hbar } \left [ - \frac{\hbar^2\Psi}{2m}\nabla^2 \Psi^* %2B U\Psi\Psi^* \right ],\\
\end{align}](/2012-wikipedia_en_all_nopic_01_2012/I/9d293db0525e23a122a08a705f74768d.png)
![\begin{align}
\frac{\partial \rho}{\partial t} & = \frac{1}{i\hbar } \left [ -\frac{\hbar^2\Psi^{*}}{2m}\nabla^2 \Psi %2B U\Psi^{*}\Psi \right ] - \frac{1}{i\hbar } \left [ - \frac{\hbar^2\Psi}{2m}\nabla^2 \Psi^{*} %2B U\Psi\Psi^{*} \right ] \\
& = \frac{\hbar}{2im} \left [ \Psi\nabla^2 \Psi^{*} - \Psi^{*}\nabla^2 \Psi \right ] \\
\end{align}](/2012-wikipedia_en_all_nopic_01_2012/I/d894d413e02f8d0137b62fb4990e646e.png)
![\begin{align} \nabla \cdot \mathbf{j} & = \nabla \cdot \left [ \frac{\hbar}{2mi} \left ( \Psi^{*} \left ( \nabla \Psi \right ) - \Psi \left ( \nabla \Psi^{*} \right ) \right ) \right ] \\
& = \frac{\hbar}{2mi} \left [ \Psi^{*} \left ( \nabla^2 \Psi \right ) - \Psi \left ( \nabla^2 \Psi^{*} \right ) \right ] \\
& = - \frac{\hbar}{2mi} \left [ \Psi \left ( \nabla^2 \Psi^{*} \right ) - \Psi^{*} \left ( \nabla^2 \Psi \right ) \right ] \\
\end{align}](/2012-wikipedia_en_all_nopic_01_2012/I/8159dc7c7c95f21b7f577568b00bd1d4.png)
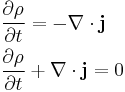
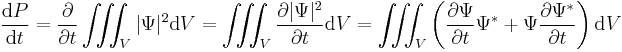
![\begin{align} & \frac{\partial \Psi}{\partial t} = \frac{i \hbar}{2m} \nabla^2 \Psi - \frac{i}{\hbar} U \Psi \\
& \frac{\partial \Psi^*}{\partial t} = - \left [ \frac{i \hbar}{2m} \nabla^2 \Psi^* - \frac{i}{\hbar} U \Psi^* \right ] \\
\end{align}\,\!](/2012-wikipedia_en_all_nopic_01_2012/I/3f7f00977c3227d8170cfb8ef577fec0.png)

![\iiint_V \frac{\partial |\Psi|^2}{\partial t} \mathrm{d}V = - \iiint_V \nabla \cdot \left [ \frac{\hbar}{2mi} \left(\Psi^* \nabla \Psi - \Psi \nabla \Psi^* \right ) \right ] \mathrm{d}V](/2012-wikipedia_en_all_nopic_01_2012/I/fd1a59d9ea7ca424ffc26159de8d9491.png)